Six Functions of a $1

'Six Functions of a $1' is a suite of computational routines commonly used in finance, economics, engineering, and real estate. In any circumstance where the time value of money is a necessary consideration for investment or return on investment, these routines play a key role in analysis and decision-making.

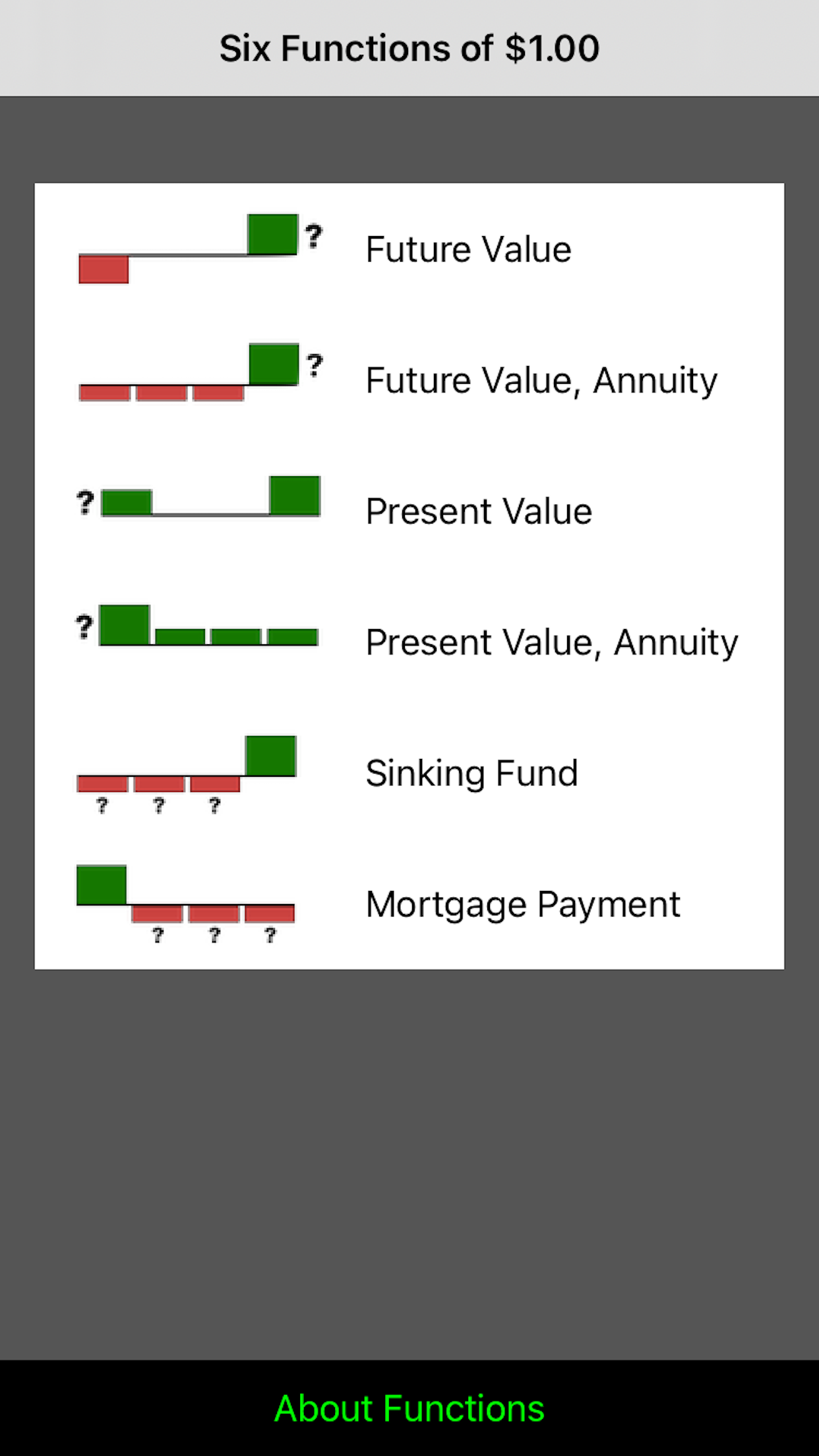
The six functions are:

• Future value
• Future value of an annuity
• Present value
• Present value of an annuity
• Sinking fund amount
• Mortgage payment amount

examples

Here are some real-world examples that highlight the use of each of the six functions:

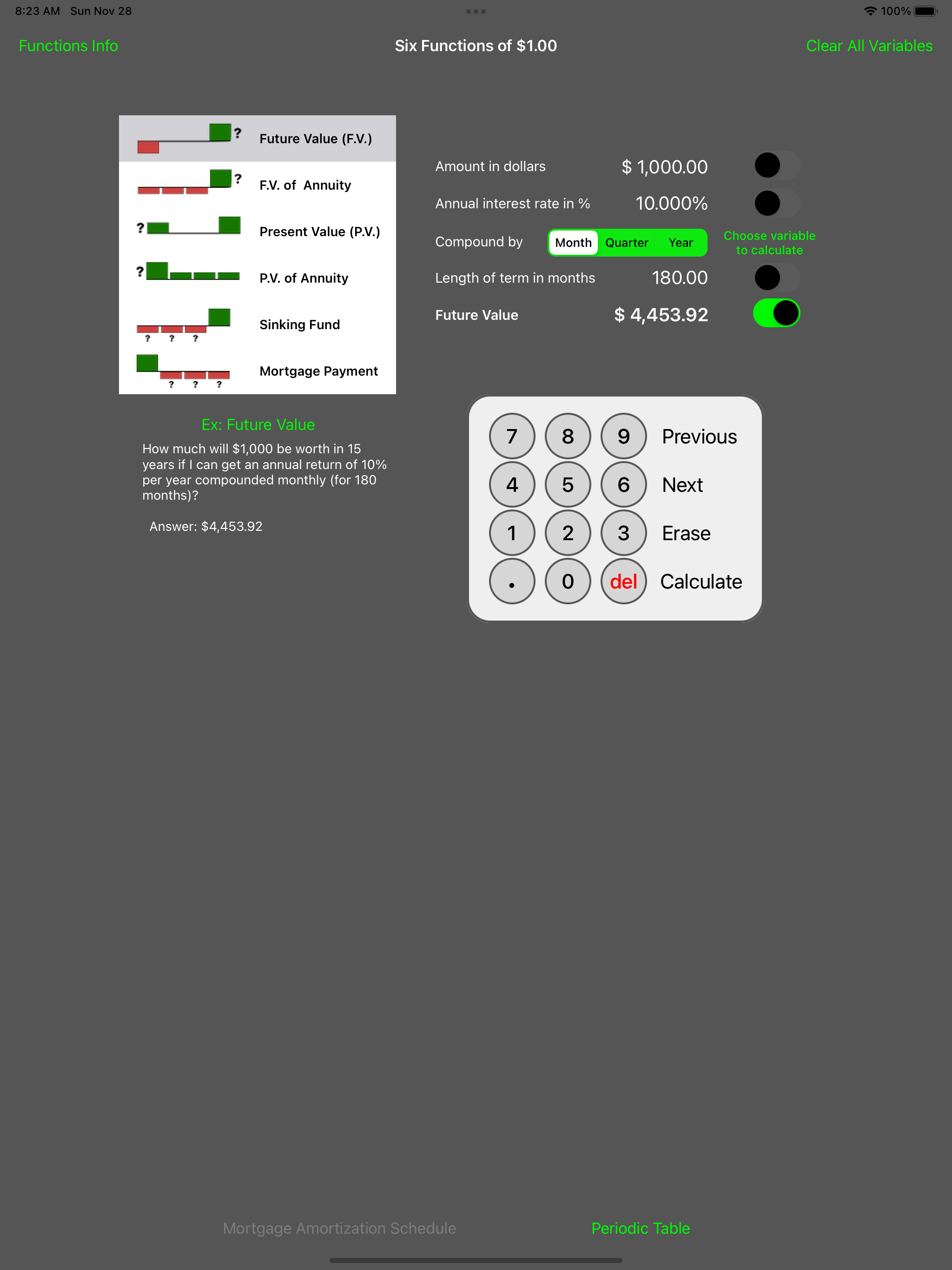
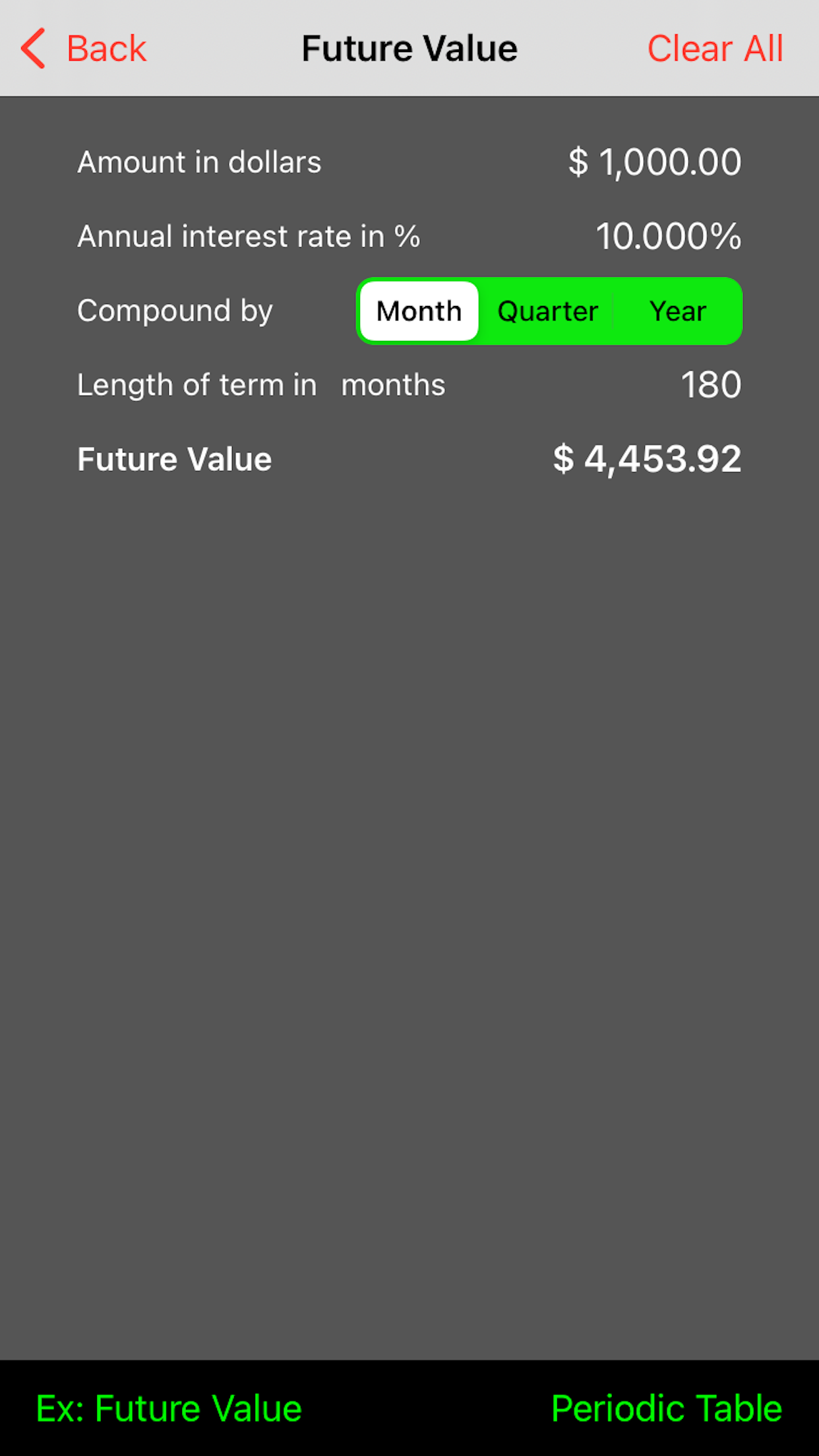
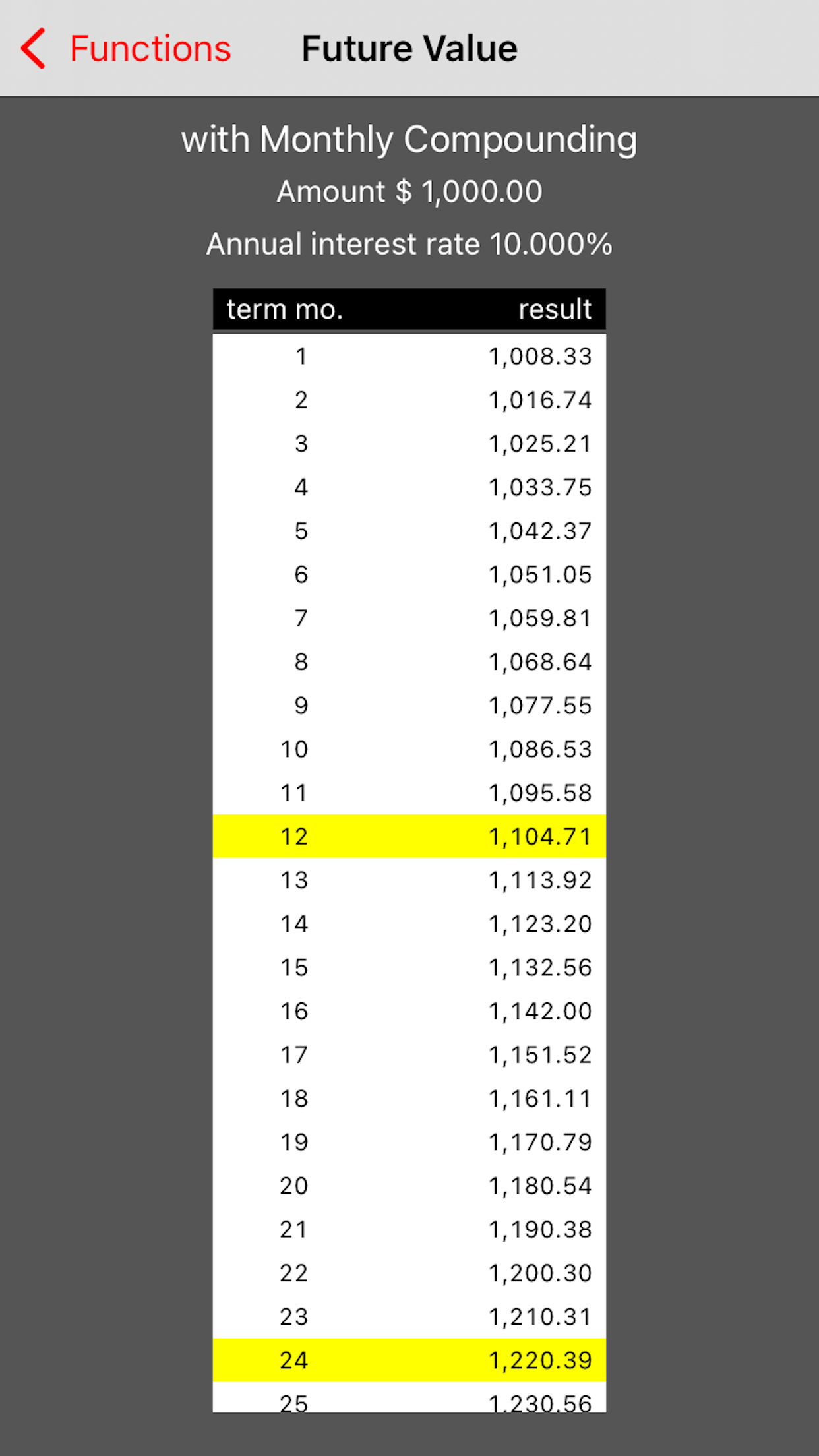
Future value:

How much will $1,000 be worth in 15 years if I can get an annual return of 10% per year compounded monthly (for 180 months)?
  Answer: $4,453.92

Future value, annuity

I'd like to save for our daughter's college education by investing $5,000 a year for the next 5 years. I can earn 6% per year on this annual annuity. How much will this plan achieve?
  Answer: $28,185.46

Present value:

I have a chance to purchase an investment that will not produce annual returns but will return $50,000 to me in 10 years. The seller is driving a hard bargain, but the investment has relatively little risk. Thus, I will apply a discount rate of just 3.5% per annum compounded monthly for this opportunity. With these assumptions, what is the maximum amount I should invest in this opportunity?
  Answer: $35,252.36

Present value, annuity:

Our daughter's grandparents are planning to put $25 per month into an existing college fund. The fund is expected to earn 4.375% per year over the next 15 years (180 months). Rather than contributing on a monthly basis, her grandparents are able to make a lump sum contribution today. How much would that lump sum contribution be to produce the same investment objective?
  Answer: $3,295.46

Sinking fund:

I own a small industrial building that has an old HVAC system that I expect to replace in 5 years at a cost of $5,000. In order to pay for the replacement, I want to set aside a small amount of money each quarter (20 total quarters) rather than pay the full amount later. A lender will pay me 8% per annum on the quarterly annuity investment. What is that quarterly amount?
  Answer: $205.78

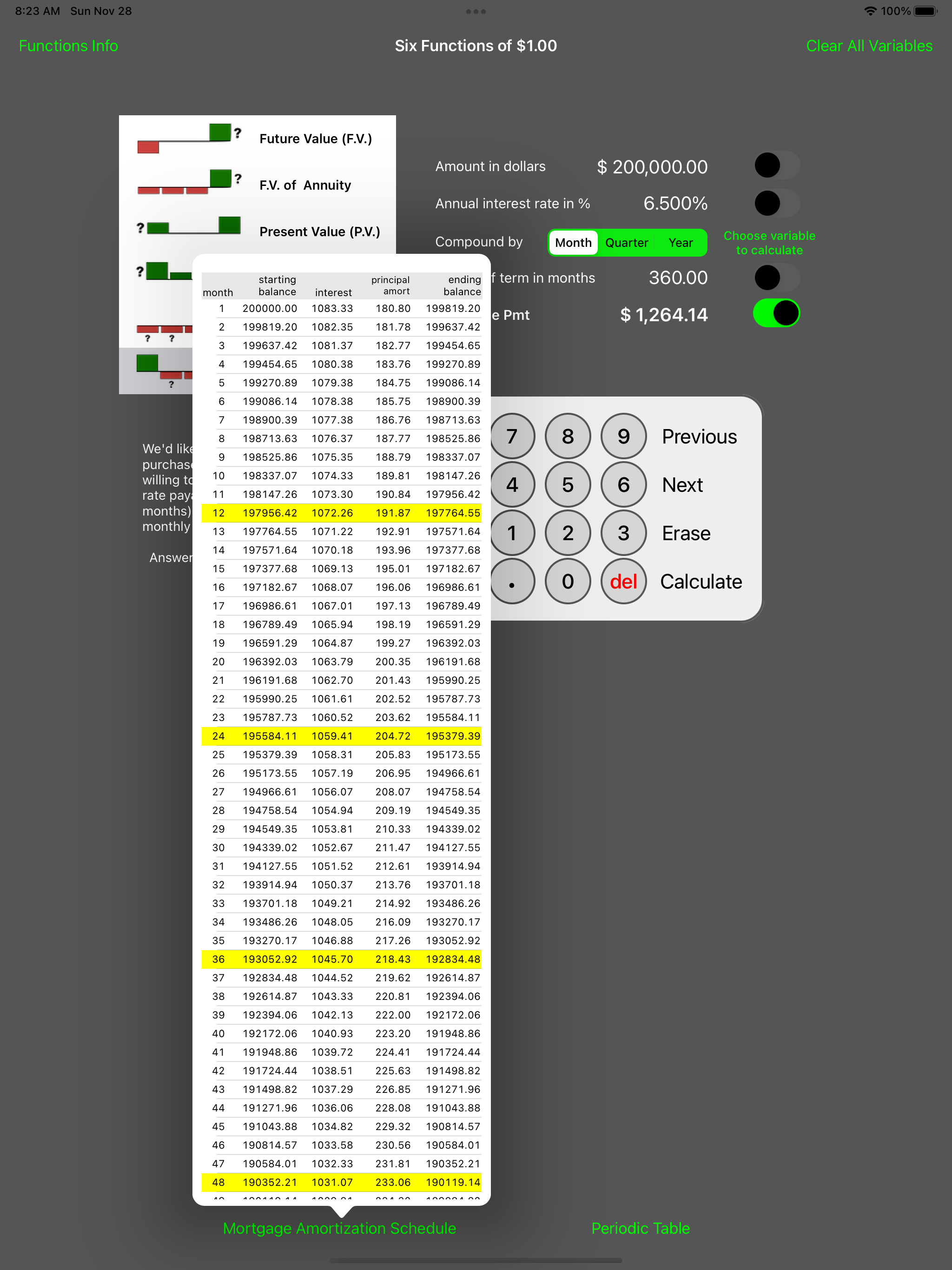
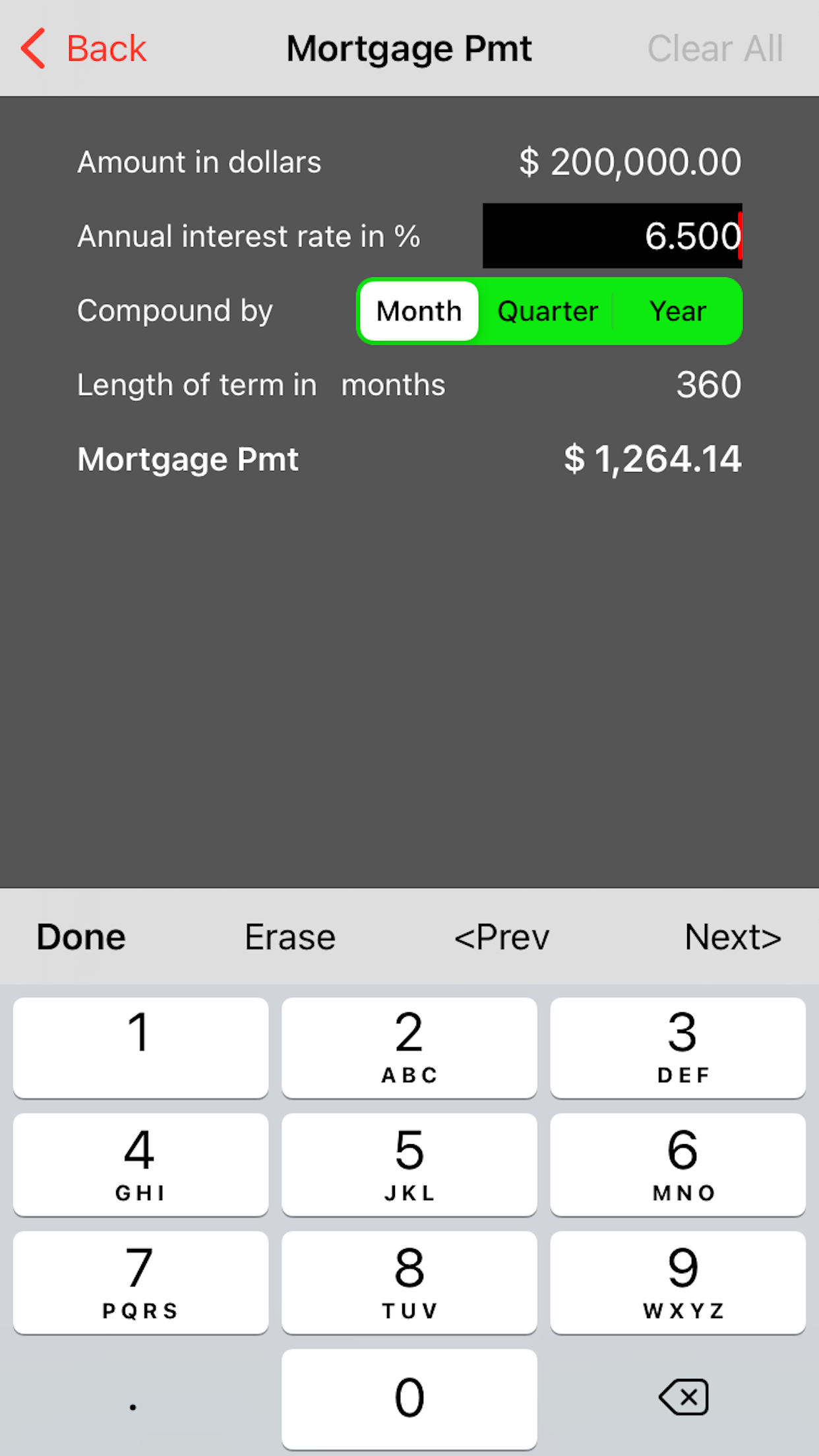
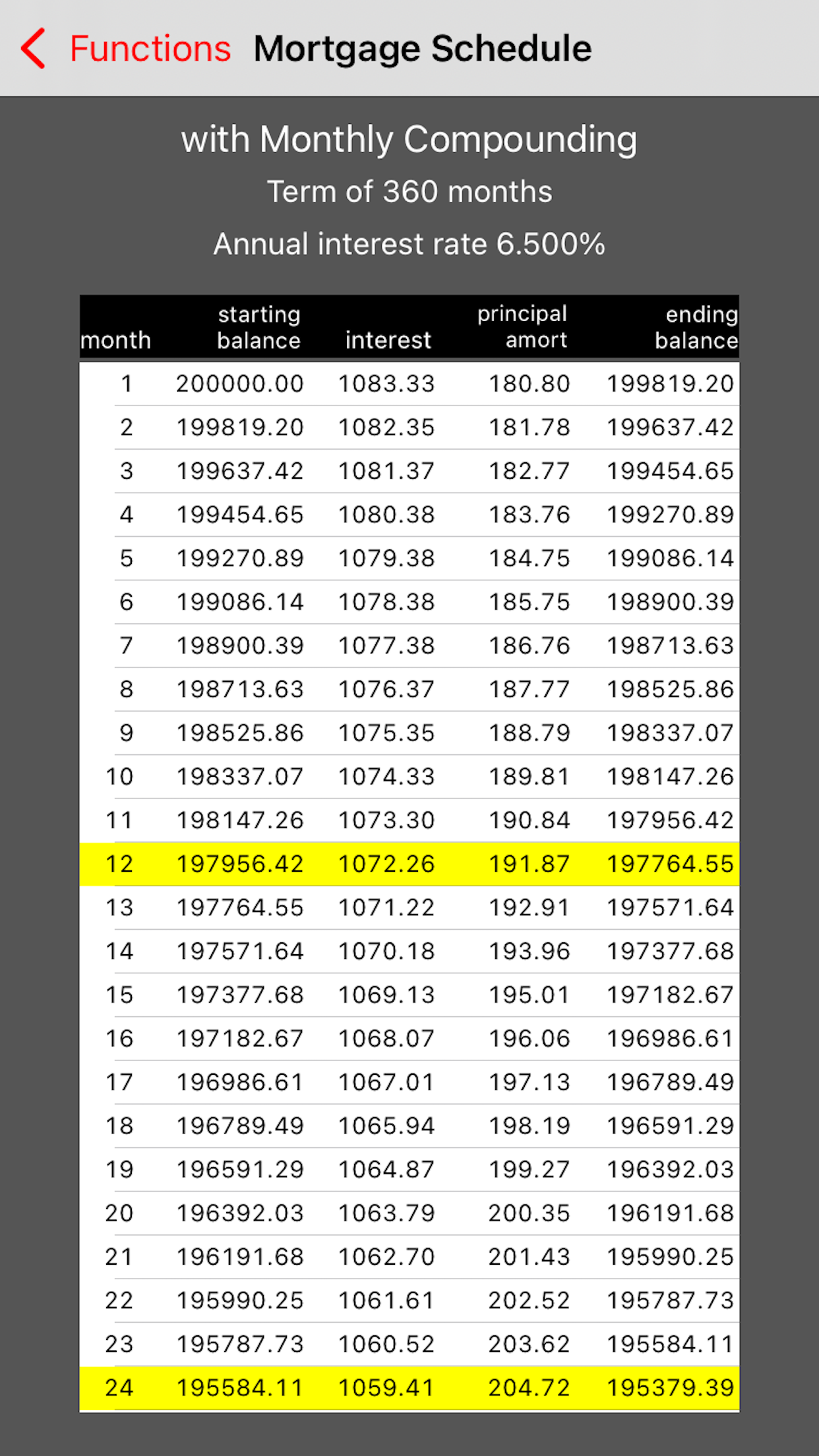
Mortgage payment:

We'd like to get a $200,000 mortgage to purchase a new home. Our lender is willing to lend at a 6.5% annual interest rate payable monthly for 30 years (360 months). On these terms, what will be our monthly mortgage payment?
  Answer: $1,264.14

changes in version 5.8

• Updated and compiled for iOS 15
• Navigation and GUI changes, especially in iPad version
• Numerous code enhancements

changes in version 5.1

• iPad version has new, floating keypad for numerical data entry
• Source code improvements

changes in version 5.0

• The iPad version has been extensively rewritten to allow selection of one of the four variables to calculate given each of the other three
• Also, the iPad version allows decimal terms in months, quarters, or years
• Custom keypad now is organized in the conventional numerical order
• Minor GUI changes

changes in version 4.0

• Rewrite and redesign for iOS 7
• Expanded number of quarters to 160
• GUI fixes

changes in version 3.0

• Major upgrade to include an iPad version
• Changes and enhancements to workflow, GUI, and functionality